Lunar Parallax
Estimating the Moon's Distance
Parallax is the apparent shift caused by viewing an object from two different vantage points. You can see it easily just by alternately blinking your left and right eye. Parallax is also evident in the apparent position of the Moon viewed from two distant points on the Earth, or from the same point six hours apart. Hipparchus, in the second century BC, derived a very good estimate of the distance to the Moon using lunar parallax.
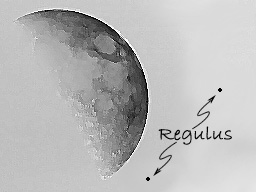
The LPOD Lunar Photo of the Day for May 26, 2007 shows the parallactic shift in the Moon's position as seen from two locations about 1400 miles apart. Amateur astronomers Anthony Ayiomamitis and Pete Lawrence (link to Pete's original page from the Internet Archive) took pictures of the Moon from Greece and England at the same moment on the afternoon of May 23. They combined the photos so that the Moon images overlap, revealing an apparent shift of about a third of a degree in the Moon's position relative to Regulus, a bright star in the constellation Leo.
I added my two cents to the discussion of this image on sci.astro.amateur, offering a refined estimate of the Moon's distance. Here, I'm exhibiting several LightWave renderings showing the geometry of the image. These use my star plotter along with a short program I wrote specifically to analyze the Moon/Regulus image, which gave me the positions and orientations of the Earth and Moon, the sunlight angle, and the sight lines to the Moon from Selsey and Athens.
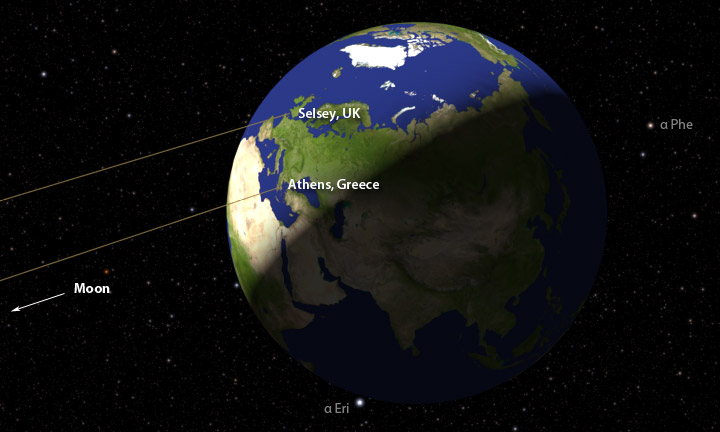
Selsey and Athens are in sunlight because the photos were taken during the day, near the time of a lunar occultation of Regulus (actually a near miss in these two locations).
Most diagrams of lunar parallax (including the one further down on this page) are wildly out of scale. This is necessary in order to make the angles visible, but I think the iconography of diagrams like these has ingrained in people a distorted sense of the scale of the solar system. The renderings below and along the left margin show the true scale of the Earth-Moon system.
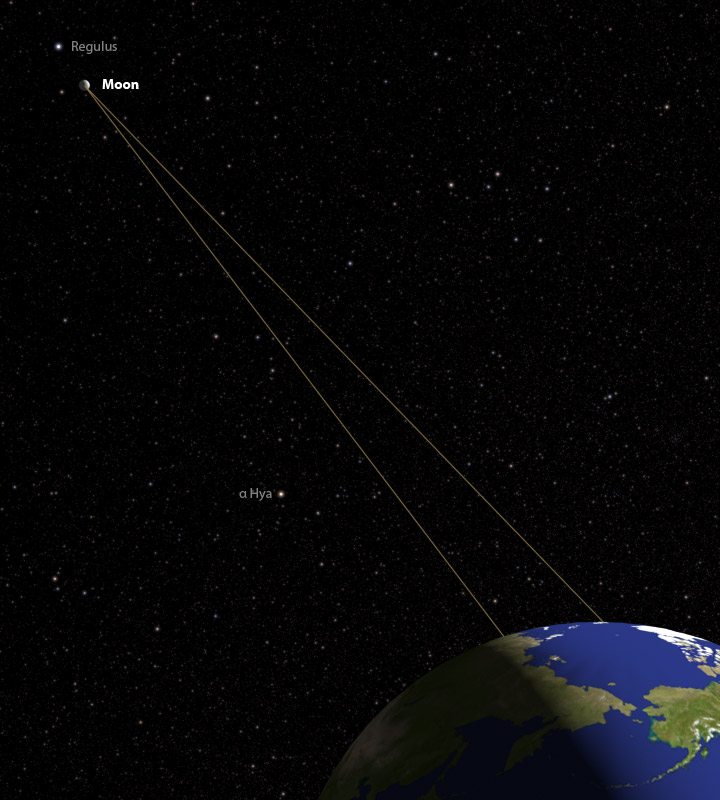
The two images below simulate the view of the Moon from Selsey (left) and Athens. Try these as a stereo pair: cross your eyes until the two Moons overlap. If you have the knack for this, the two Moons will appear to fuse into a single 3D image.

Selsey, UK

Athens, Greece
With a little geometry, the parallax in these views can be used to estimate the distance to the Moon.
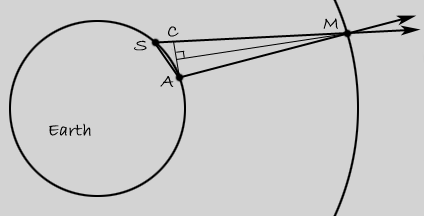
Selsey, Athens, and the Moon (S, A, and M in the diagram) form a long, thin triangle. The angle at M is the parallax, the angular separation of the two images of Regulus, about 1100 arcseconds. We also know the length of side AS, which is just the chord distance between Athens and Selsey, about 2360 kilometers.
If we make the simplifying assumption that triangle ASM is isosceles (that SM and AM are the same length), then the distance to the Moon is just
( length of AS ) / ( 2 tan( parallax / 2 ))
Plugging in 2360 kilometers and 1100 arcseconds gives us an estimate of the lunar distance that's too large by about 13%. We can do much better by finding the length of the line AC and using that instead of AS in the formula. One way to estimate AC's length, thinking like a computer graphics person, is to create unit direction vectors for AS and AM. The dot product of these two vectors is the cosine of the angle at A. The sine of this angle is the foreshortening of AS in the direction of M, or the length of the projection of AS onto the image plane, and this is the length of AC, or near enough.
A related approach is to use the angle at A (or S), the length of AS, and the parallax (the angle at M) to solve the scalene triangle directly.
length of MS = ( length of AS ) sin( angle at A ) / sin parallax
length of MA = ( length of AS ) sin( angle at S ) / sin parallax
Either way, we get within an eyelash of the Moon's true distance from the observing sites. But using 2360 kilometers and 1100 arcseconds gives us a value that's too small by about 4%. What happened?
Well, at this point we can cheat: the true parallax is more like 1056 arcseconds, a difference that amounts to about 15 pixels on the composite image Anthony created. The simplest explanation for the discrepancy is that Pete and Anthony didn't take their pictures at exactly the same time. A difference of a minute and a half would be enough, since the Moon moves its own width (half a degree) every hour. Another possibility is that they were farther apart than I've assumed, but the extra distance would need to be about a hundred kilometers in order to account for the entire discrepancy.
The methods of early Greek astronomers have a similar flavor. We don't know Hipparchus's exact procedure, but we do know that he used an estimate of the lunar parallax derived from a solar eclipse. The eclipse was total at the Hellespont. At Alexandria, the Moon covered 4/5 of the Sun's diameter. The parallax was therefore 1/10 of a degree, and the baseline was the distance from Alexandria to the Hellespont. Hipparchus had to assume that maximum eclipse occurred at the same time in the two locations and that the Sun's parallax was imperceptibly small, and he needed a good estimate of the size of the Earth.
Three centuries later, Ptolemy laid out a detailed explanation of the geometry of the problem in book V, section 13 of his Almagest. He even included a diagram very similar to the one here. Ptolemy's figures for the Moon's distance are encumbered by a lunar theory and supporting arguments that aren't merely wrong, they're intellectually dishonest, at least by modern standards. But if we give him the benefit of the doubt, his estimate of 59 Earth radii at the syzygies (new and full Moon) is comfortably in the middle of the modern range of values for the Moon's elliptical orbit, roughly 56 to 64 e.r.
The parallax method couldn't be extended to the planets or the stars until the invention of the telescope. In the 1990's, the Hipparcos satellite used it to measure distances of up to a thousand light-years, about 25 million times the distance to the Moon. Meanwhile, by bouncing laser light off the reflectors left on the Moon's surface by Apollo astronauts, we can routinely measure the distance to the Moon with an accuracy of a few centimeters.

